[最も共有された！ √] (x y)^3 expansion 103862

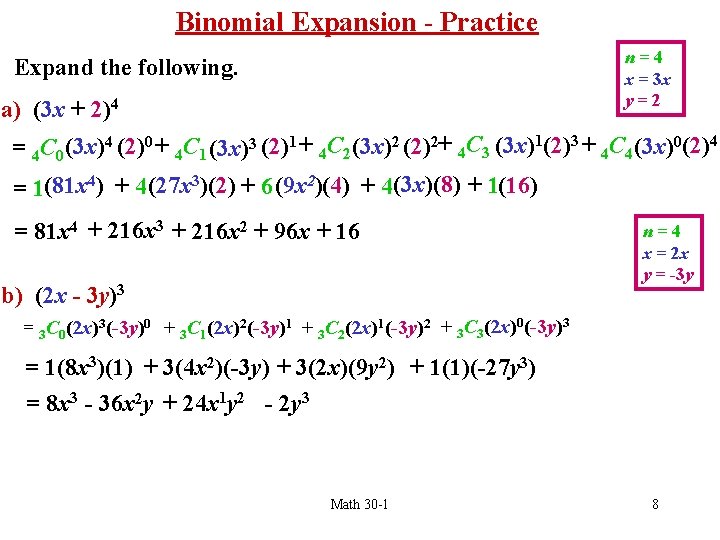
243x 5 810x 4 y 1080x 3 y 2 7x 2 y 3 240xy 4 32y 5 Finding the k th term Find the 9th term in the expansion of (x2y) 13 Since we start counting with 0, the 9th term is actually going to be when k=8 That is, the power on the x will 138=5 and the power on the 2y will be 8 The expansion is y^55y^4x10y^3x^210y^4x^35y^5x^4x^5 We need to use Pascal's Triangle, shown in the picture below, for this expansion Because the binomial is raised to the 5th power, we need to use the 5th row of the triangle Expansion of (xy) 3 2 See answers 9304gaurikatrehan9c 9304gaurikatrehan9c Answer 3x3y is the ans of ur question naveena75 naveena75 Answer 3×x3×y hope it helps u New questions in Math sazoitvdgqgirls come here

Myriorama Cards Were Invented In France Around 13

(x y)^3 expansion

(x y)^3 expansion-In the denominator for each term in the infinite sum History⋅(1)3−k ⋅(−x)k ∑ k = 0 3 ⁡ 3!

Myriorama Cards Were Invented In France Around 13

La calculadora puede realizar expansiones logarítmicas de expresión de la forma ln (a*b), dando los resultados en forma exacta así para expandir ln ( 3 ⋅ x), se debe ingresar expansion_log ( ln ( 3 ⋅ x)) , después del cálculo, se devuelve el resultadoThe following are algebraix expansion formulae of selected polynomials Square of summation (x y) 2 = x 2 2xy y 2 Square of difference (x y) 2 = x 2 2xy y 2 Difference of squares x 2 y 2 = (x y) (x y) Cube of summation (x y) 3 = x 3 3x 2 y 3xy 2 y 3 Summation of two cubes x 3 y 3 = (x y) (x 2 xy y 2) CubeQuestion Identify the binomial expansion of (xy)^3 Answer by rapaljer (4671) ( Show Source ) You can put this solution on YOUR website!

The above expansion holds because the derivative of e x with respect to x is also e x, and e 0 equals 1 This leaves the terms (x − 0) n in the numerator and n!In the expansion of (5 x 3 y) n, (5 x 3 y) n, each term has the form (n k) a n – k b k (n k) a n – k b k, where k k successively takes on the value 0, 1, 2,, n 0, 1, 2,, n If (n k) = (7 2), (n k) = (7 2), what is the corresponding term?Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!

Rearranging the terms in the expansion, we will get our identity for x 3 y 3 Thus, we have verified our identity mathematically Again, if we replace x with − y in the expression, we haveIn elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomialAccording to the theorem, it is possible to expand the polynomial (x y) n into a sum involving terms of the form ax b y c, where the exponents b and c are nonnegative integers with b c = n, and the coefficient a of each term is a specific positive6 What is the coefficient of x7 in (1 x)11?

The Binomial Expansion Of X Y22 Is A X2 2xy2 Y4 Gauthmath

Solved Find The Coefficient Of X 4 Y 5 In The Expansion Of Chegg Com

Answer (1 of 5) First of all, we observe the following formula {{\left( a\,\,b \right)}^{\,3}}\,=\,{{a}^{\,3}}\,\,{{b}^{\,3}}\,\,3\,a\,b\,\left( a\,\,b \rightThe number of terms in the expansion of x4y 3 x4y 3 2 is 6 7 8 12 x 4y 3 x – 4y 3 2 = x 2 – 4y 2 6 = x 2 – 16y 2 6No of terms in the expansioFree expand & simplify calculator Expand and simplify equations stepbystep

Online Tutoring On Maths Binomial Theorem

Print Tolerance Tuning Horizontal Xy Expansion Duet3d

X^3 y^3 z^3 3x^2y 3xy^2 3x^2z 3z^2x 3y^2z 3z^2y 6xyz Lennox Obuong Algebra Student Email obuong3@aolcomBinomial Expansions Binomial Expansions Notice that (x y) 0 = 1 (x y) 2 = x 2 2xy y 2 (x y) 3 = x 3 3x 3 y 3xy 2 y 3 (x y) 4 = x 4 4x 3 y 6x 2 y 2 4xy 3 y 4 Notice that the powers are descending in x and ascending in yAlthough FOILing is one way to solve these problems, there is a much easier way a^3 3a^2b 3ab^2 b^3 Use the Binomial expansion (note the exponents sum to the power in each term) (xy)^3 = _3C_0x^3y^0 _3C_1x^2y^1 _3C_2x^1y^2 _3C_3x^0y^3

Myriorama Cards Were Invented In France Around 13

Expanding Binomials Video Series Khan Academy

The coefficient of x^y in the expansion of (2x Cheggcom Math Advanced Math Advanced Math questions and answers 1 The coefficient of x^y in the expansion of (2x 3y)10 is 2 The constant term in the expansion of (2x 3)° is Question 1 The coefficient of x^y in the expansion of (2x 3y)10 is 2The coefficient of x2y3 in the expansion of (1 −x y) is 2!3!! Binomial Theroem 0 2299 6 535 Find the coefficient of x^3 y^3 z^2 in the expansion of (xyz)^8 MathCuber 0 users composing answers

15 Pokemon Xy Roaring Skies Expansion Set Reverse Foil Wurmple 3 M0p Ebay

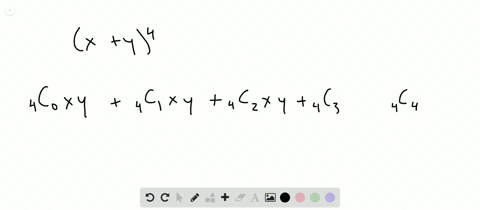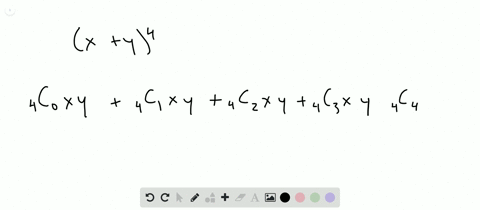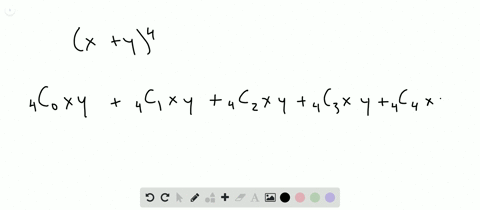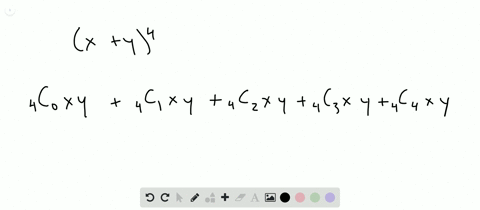
Solved Find The Expansion Of X Y 4 A Using Combinatorial Reasoning As In Example 1 B Using The Binomial Theorem

You can put this solution on YOUR website!Transcribed image text Coefficient of themal expansion versus temperature forsteel 2 X Y 3 Temp (°F) Coefficient of thermal expansion, a 4 80 647E06 5 612E06 6 0 7 600E06 586E06 572E06 8 40 9 60 558E06 10 80 543 E06 11 100 528E06 12 1 509E06 13 180 452 E06 0 15 16 2 240 17 430E06 408E06 3E06 358E06 276E06 245E06⋅(X)4−k ⋅(Y)k ∑ k = 0 4 ⁡

12 Expansion Of X Y 3 Y Z 3 Z X 3 Is A 2x 3 2y

X Y 2 3 Find The Expansion Of The Following Brainly In

1234567891011Next